Quantum physics already feels like a puzzle, but now scientists are becoming more literal. A team of mathematicians at the University of Colorado at Boulder has designed a quantum Rubik’s Cube with endless possibilities and strange new movements that can be used to solve it.
The classic (and classic) Rubik’s Cube is what is called a permutation puzzle, and certain actions must be performed to reposition any of the many possible permutations into a “resolved” state.
For the infamous cube, it’s around 43 Kintirion possible The combination of small blocks of color is consistently categorized into six faces of color through a series of constrained movements.
However, quantum Rubik’s cube crank takes up limitless space. All you need to do is give the solver a new quantum action. This is the ability to move the work into the superposition of quantums that move it. It’s not moved at the same time.
“The overlapping means that unlike common permutation puzzles from toy stores, the number of unique permitted states for puzzles is endless.” Write it in their paper.
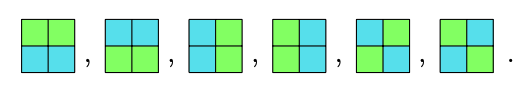
The team tested the idea with a simple version of the permutation puzzle. It is a two-dimensional 2×2 grid, composed exclusively of blue and green tiles. The resolved state was to place two blue tiles on top of two blue tiles.
In its classic form, the puzzle has only six possible permutations containing the resolved state. Any state can be converted to other states via a series of sequences that exchange vertical and horizontal tiles. It is prohibited to replace diagonal tiles to rotate the entire puzzle.
This basic puzzle can give it a quantum flavor by calling “particles” of color and pointing out that each tile is in a way intertwined, as it is indistinguishable from other tiles of the same color.
“Particles” have a quantum touch, but in reality the puzzle itself is still played using classical movements. If superposition between two different particles is allowed, the true quantum version opens.
Three different types of simulated players worked to solve puzzles from 2,000 random scrambles. The only movement of the classic solver was to exchange two adjacent tiles. Quantum Solver could only enter pairs for quantum superposition. And the total solver can perform either action each time.
Naturally, the combined solver performed best, solving the puzzle with an average movement of 4.77. Quantum Solver then appeared in the final place with an average movement of 5.32 and the Classic Solver with an average movement of 5.88.
That does not mean that the field of classical physics has no advantages. Classic solvers actually get to the solution with less than 5 moves more frequently than Quantum Solver. But it blows that average. Because it often takes twice as long, so Quantum Solver almost always finishes with less than 8.
This so-called quantum advantage should become more pronounced in more complex puzzles, the team says.
After the solver operates using permutations using permitted movements (classic, quantum, or both), the solution is verified via “judgment”.
If you are familiar with the old Schrödinger cat thinking experiments, you will remember that the measurement itself is just one of the states, randomly the superposition. Ideally, it’s a resolved state, but if not, the puzzle will be scrambled again and the solver will have to be redoed.
This is how Classic Solvers begin working on quantum puzzles. Unless they are very fortunate and the scrambling state is one of six classic possibilities (from the infinite quantum option), they must move as close as possible to the solution, hoping that the measurement will disrupt the superposition to the resolved state.
While Quantum Solver appears to have the advantages of home ground, it has one drawback. Two movements are required to perform a classic swap operation. This is how classic solvers get early head start in some versions of the puzzle, but why combined solvers have leads.
The team also created a 3D version of the quantum puzzle, although not a full cube. It was a 2x2x1 tile, which also had infinite possibilities and was solved with similar actions.
In practice, it is possible to construct a quantum forward puzzle using an array of ultra-cold atoms suspended in an optical lattice. But most of the time, it’s a thought experiment for a mathematics nerd.
This study has been accepted as published in a journal Physical Review aand is currently available on Preprint Server arxiv.







